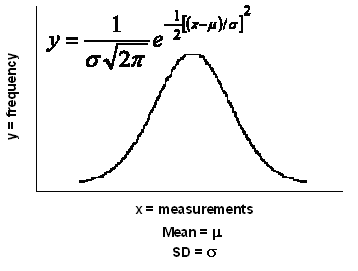The ancient Phoenicians were great traders and sea farers, but they tended to
overload their boats. In stormy weather, goods had to be thrown overboard in
order to save the ship. Owners of lost goods were then compensated by those who
did not lose their goods, and the amounts involved depended on the total value
of the goods. This arrangement was named havara, and this term evolved over the
centuries to become average, and from average the mathematical term mean developed.
The astronomer, Gauss, measured distances between stars. He noticed that it
was difficult to reproduce his measurements exactly. However, the measurements
clustered around a central value, more common near the mean, and becoming less
common as they are further away from the mean (fig. left).
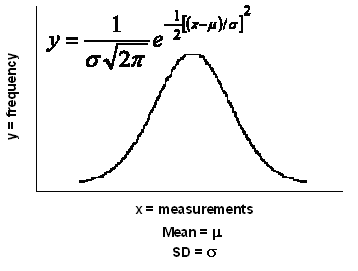
He concluded that any set of
measurements would normally distribute to this pattern and called it the
Normal Distribution.
De Moivre derived the formula for the Normal Distribution curve in
mathematical terms (fig. right).

Once this was done, the features of Normal Distribution could be
mathematically handled. Using simple calculus, the area under the curve (or any
part of it) can be estimated. Fisher developed the concept further and used the
area under the curve as a measure of probability. He argues that if the area
under the whole curve be consider totality, then any portion represents the
probability of the events that describe the portion. From this, he was able to
calculate the probability of obtaining a measurement that exceeds a deviation
from the mean value. He standardized the measurement of this deviation and
called it the Normal Standard Deviate (z), later abbreviated to Standard
Deviation, and derived the relationships between z and probability.
The Standard Deviation z and its probability are the basis of many statistical
calculations, including differences between groups and sample size
estimations. However these calculations are often embedded into
computer programs and are not visible.
A few z values are however much more commonly used, the most frequent one
being the z value of 1.96, as the probability of having a value exceeding this
is 0.025 (2.5%), so that 95% confidence intervals in any Normally distributed
measurement is mean ± 1.96 SDs.
Example 1
Example 2
We understand that, for the IQ, the population mean is 100
and the Standard Deviation is 10. What is the proportion of the population
that has an IQ of 120 or more.
The answer is as follows. The IQ 120 is 20 points (2 Standard Deviations)
above the mean, and the probability of z>=2 is 0.023, so 2.3%. In other words,
an IQ of 120 is 97.7 percentile.
If population mean and SD for IQ is 100 and 10, what IQ levels
can be considered abnormal.
The argument is as follows. If we accept normality as the range within which 95%
of the population can be found, then abnormal values must be the 5% outside of
this range. Assuming a symmetrical normal distribution, this means 2.5% on
each side of the normal distribution curve. As the z value for p=0.025 is 1.96,
then those with IQ 1.96 SD less than the mean (100 - 1.96*10 = 100 - 19.6 = 80.4)
can be considered subnormal at 2.5 percentile, and those with IQ 1.96 SD more
than the mean (100 + 1.96*10 = 100 + 19.6 = 119.6) can be considered supernormal
at 97.5 percentile.
Common z Values
Table of Probability of z
| Probability | z value |
|---|
| 0.20 | 0.85 | * this is often used in in power calculations power=0.8, β=0.2 |
| 0.10 | 1.29 |
| 0.05 | 1.65 | * this is often used for testing statistical significane p<0.05 |
| 0.025 | 1.96 | * this is often used to define the 95% confidence interval (2.5% each side) |
| 0.01 | 2.33 |
| 0.005 | 2.58 |
| 0.0025 | 2.81 |
| 0.001 | 3.10 |
The z value is the sum of the first row and first column, and probability is the cell of that row and column.
for example, probability=0.40905 when z=0.23
Please be reminded that, although probabilities of z are presented
to 5 decimal points of precision in the table, only a 2-4 decimal point precisions
are more commonly used.
| 0.00 | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 | 0.06 | 0.07 | 0.08 | 0.09 |
|---|
| 0.0 | 0.50000 | 0.49601 | 0.49202 | 0.48803 | 0.48405 | 0.48006 | 0.47608 | 0.47210 | 0.46812 | 0.46414 |
|---|
| 0.1 | 0.46017 | 0.45620 | 0.45224 | 0.44828 | 0.44433 | 0.44038 | 0.43644 | 0.43251 | 0.42858 | 0.42465 |
|---|
| 0.2 | 0.42074 | 0.41683 | 0.41294 | 0.40905 | 0.40517 | 0.40129 | 0.39743 | 0.39358 | 0.38974 | 0.38591 |
|---|
| 0.3 | 0.38209 | 0.37828 | 0.37448 | 0.37070 | 0.36693 | 0.36317 | 0.35942 | 0.35569 | 0.35197 | 0.34827 |
|---|
| 0.4 | 0.34458 | 0.34090 | 0.33724 | 0.33360 | 0.32997 | 0.32636 | 0.32276 | 0.31918 | 0.31561 | 0.31207 |
|---|
| 0.5 | 0.30854 | 0.30503 | 0.30153 | 0.29806 | 0.29460 | 0.29116 | 0.28774 | 0.28434 | 0.28096 | 0.27760 |
|---|
| 0.6 | 0.27425 | 0.27093 | 0.26763 | 0.26435 | 0.26109 | 0.25785 | 0.25463 | 0.25143 | 0.24825 | 0.24510 |
|---|
| 0.7 | 0.24196 | 0.23885 | 0.23576 | 0.23270 | 0.22965 | 0.22663 | 0.22363 | 0.22065 | 0.21770 | 0.21476 |
|---|
| 0.8 | 0.21186 | 0.20897 | 0.20611 | 0.20327 | 0.20045 | 0.19766 | 0.19489 | 0.19215 | 0.18943 | 0.18673 |
|---|
| 0.9 | 0.18406 | 0.18141 | 0.17879 | 0.17619 | 0.17361 | 0.17106 | 0.16853 | 0.16602 | 0.16354 | 0.16109 |
|---|
| 0.00 | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 | 0.06 | 0.07 | 0.08 | 0.09 |
|---|
| 1.0 | 0.15866 | 0.15625 | 0.15386 | 0.15151 | 0.14917 | 0.14686 | 0.14457 | 0.14231 | 0.14007 | 0.13786 |
|---|
| 1.1 | 0.13567 | 0.13350 | 0.13136 | 0.12924 | 0.12714 | 0.12507 | 0.12302 | 0.12100 | 0.11900 | 0.11702 |
|---|
| 1.2 | 0.11507 | 0.11314 | 0.11123 | 0.10935 | 0.10749 | 0.10565 | 0.10383 | 0.10204 | 0.10027 | 0.09853 |
|---|
| 1.3 | 0.09680 | 0.09510 | 0.09342 | 0.09176 | 0.09012 | 0.08851 | 0.08691 | 0.08534 | 0.08379 | 0.08226 |
|---|
| 1.4 | 0.08076 | 0.07927 | 0.07780 | 0.07636 | 0.07493 | 0.07353 | 0.07214 | 0.07078 | 0.06944 | 0.06811 |
|---|
| 1.5 | 0.06681 | 0.06552 | 0.06426 | 0.06301 | 0.06178 | 0.06057 | 0.05938 | 0.05821 | 0.05705 | 0.05592 |
|---|
| 1.6 | 0.05480 | 0.05370 | 0.05262 | 0.05155 | 0.05050 | 0.04947 | 0.04846 | 0.04746 | 0.04648 | 0.04551 |
|---|
| 1.7 | 0.04457 | 0.04363 | 0.04272 | 0.04182 | 0.04093 | 0.04006 | 0.03920 | 0.03836 | 0.03754 | 0.03673 |
|---|
| 1.8 | 0.03593 | 0.03515 | 0.03438 | 0.03363 | 0.03288 | 0.03216 | 0.03144 | 0.03074 | 0.03005 | 0.02938 |
|---|
| 1.9 | 0.02872 | 0.02807 | 0.02743 | 0.02680 | 0.02619 | 0.02559 | 0.02500 | 0.02442 | 0.02385 | 0.02330 |
|---|
| 0.00 | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 | 0.06 | 0.07 | 0.08 | 0.09 |
|---|
| 2.0 | 0.02275 | 0.02222 | 0.02169 | 0.02118 | 0.02068 | 0.02018 | 0.01970 | 0.01923 | 0.01876 | 0.01831 |
|---|
| 2.1 | 0.01786 | 0.01743 | 0.01700 | 0.01659 | 0.01618 | 0.01578 | 0.01539 | 0.01500 | 0.01463 | 0.01426 |
|---|
| 2.2 | 0.01390 | 0.01355 | 0.01321 | 0.01287 | 0.01255 | 0.01222 | 0.01191 | 0.01160 | 0.01130 | 0.01101 |
|---|
| 2.3 | 0.01072 | 0.01044 | 0.01017 | 0.00990 | 0.00964 | 0.00939 | 0.00914 | 0.00889 | 0.00866 | 0.00842 |
|---|
| 2.4 | 0.00820 | 0.00798 | 0.00776 | 0.00755 | 0.00734 | 0.00714 | 0.00695 | 0.00676 | 0.00657 | 0.00639 |
|---|
| 2.5 | 0.00621 | 0.00604 | 0.00587 | 0.00570 | 0.00554 | 0.00539 | 0.00523 | 0.00508 | 0.00494 | 0.00480 |
|---|
| 2.6 | 0.00466 | 0.00453 | 0.00440 | 0.00427 | 0.00415 | 0.00402 | 0.00391 | 0.00379 | 0.00368 | 0.00357 |
|---|
| 2.7 | 0.00347 | 0.00336 | 0.00326 | 0.00317 | 0.00307 | 0.00298 | 0.00289 | 0.00280 | 0.00272 | 0.00264 |
|---|
| 2.8 | 0.00255 | 0.00248 | 0.00240 | 0.00233 | 0.00226 | 0.00219 | 0.00212 | 0.00205 | 0.00199 | 0.00193 |
|---|
| 2.9 | 0.00187 | 0.00181 | 0.00175 | 0.00169 | 0.00164 | 0.00159 | 0.00154 | 0.00149 | 0.00144 | 0.00139 |
|---|
| 0.00 | 0.01 | 0.02 | 0.03 | 0.04 | 0.05 | 0.06 | 0.07 | 0.08 | 0.09 |
|---|
| 3.0 | 0.00135 | 0.00131 | 0.00126 | 0.00122 | 0.00118 | 0.00114 | 0.00111 | 0.00107 | 0.00103 | 0.00100 |
|---|
| 3.1 | 0.00097 | 0.00094 | 0.00090 | 0.00087 | 0.00084 | 0.00082 | 0.00079 | 0.00076 | 0.00074 | 0.00071 |
|---|
| 3.2 | 0.00069 | 0.00066 | 0.00064 | 0.00062 | 0.00060 | 0.00058 | 0.00056 | 0.00054 | 0.00052 | 0.00050 |
|---|
| 3.3 | 0.00048 | 0.00047 | 0.00045 | 0.00043 | 0.00042 | 0.00040 | 0.00039 | 0.00038 | 0.00036 | 0.00035 |
|---|
| 3.4 | 0.00034 | 0.00032 | 0.00031 | 0.00030 | 0.00029 | 0.00028 | 0.00027 | 0.00026 | 0.00025 | 0.00024 |
|---|
| 3.5 | 0.00023 | 0.00022 | 0.00022 | 0.00021 | 0.00020 | 0.00019 | 0.00019 | 0.00018 | 0.00017 | 0.00017 |
|---|
| 3.6 | 0.00016 | 0.00015 | 0.00015 | 0.00014 | 0.00014 | 0.00013 | 0.00013 | 0.00012 | 0.00012 | 0.00011 |
|---|
| 3.7 | 0.00011 | 0.00010 | 0.00010 | 0.00010 | 0.00009 | 0.00009 | 0.00009 | 0.00008 | 0.00008 | 0.00008 |
|---|
| 3.8 | 0.00007 | 0.00007 | 0.00007 | 0.00006 | 0.00006 | 0.00006 | 0.00006 | 0.00005 | 0.00005 | 0.00005 |
|---|
| 3.9 | 0.00005 | 0.00005 | 0.00004 | 0.00004 | 0.00004 | 0.00004 | 0.00004 | 0.00004 | 0.00003 | 0.00003 |
|---|
Abramowitz M and Stegun IA (1972) Handbook of mathematical functions.
Dover Publications, Inc. New York. Library of Congress Catalog card
number 65-12253. p.925